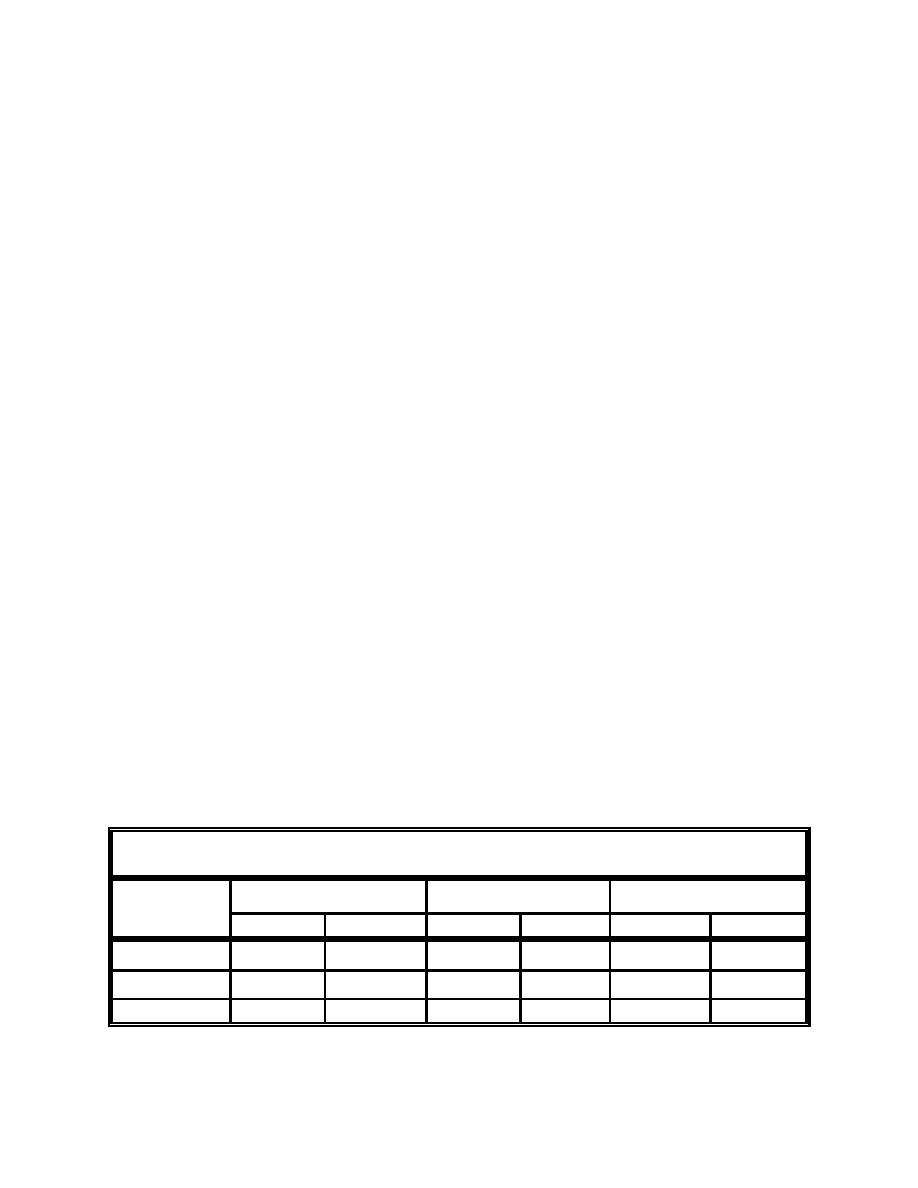
Table H1. Lengths Associated with Generic-Capped Mound in Figure H3

where

VM = volume of cap material over dredged material mound

VCA = volume of material in cap apron

VT = total volume of cap material

tpc = thickness of primary cap

tta = thickness of cap at toe of mound apron

tc = change in cap thickness over apron (tpc - tta)

r1 , r2 = long, short radius of ellipse

= subscript for dredged material mound

= subscript for inner dredged material mound (crest, inner flank and

outer flank)

= subscript for total capped surface

m = slope of change in cap thickness (i.e., 1:100!m=0.01)

The volume of cap material overlying the inner and outer flanks may be

calculated as part of the overall dredged material mound cap volume calcula-

tions. When there is no change in cap thickness over the mound apron as in

Figure H1, the cap volume over the mound apron may also be included in the

overall dredged material mound cap volume calculations. To demonstrate,

assume a generic circular mound having a relief of 2.1 m (7 ft) with cap 0.9 m

(3 ft) thick is created (Figure H3). Approximate average inner flank, outer flank,

and apron slopes are 1:50, 1:400, and 1:2000, respectively. Table H1 shows that

for this example, the horizontal length and slope length are nearly equal, so use

of the horizontal length in cap volume calculation is justified. For steeper slopes

and/or higher mound relief, this assumption should be verified.

Table H1

Lengths Associated with Generic-Capped Mound in Figure H3

Vertical Length

Horizontal Length

Slope Length

A - B Inner Flank

0.9

150

46.009

150.03

B - C Inner Flank

0.9

366

1,200

366.0011

1,200.00375

C - D Apron

0.3

610

2,000

610.000074

2,000.00025

Appendix H Level-Bottom Capping Projects