 |
||
|
|
||
| |||||||||||||||
|
|
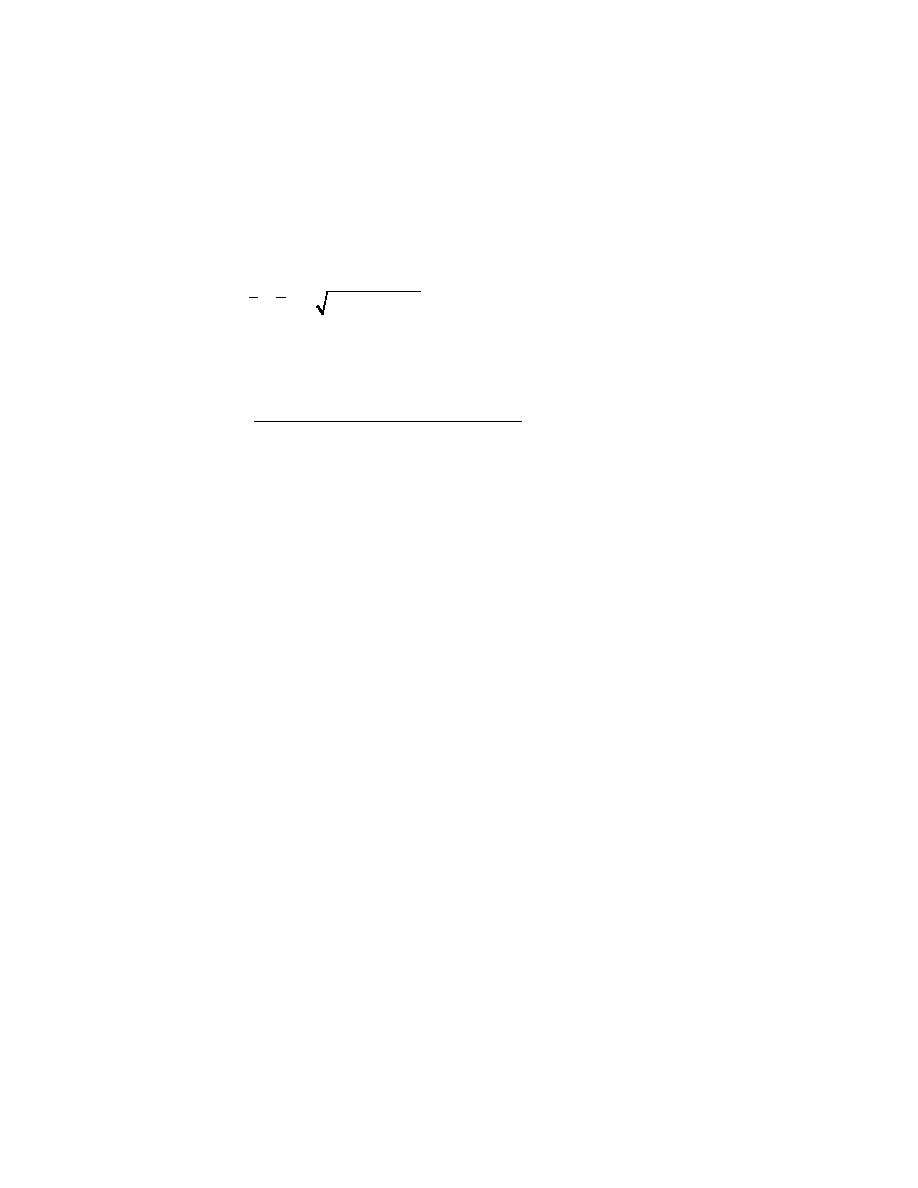 2
2
s pooled (1/ n1 + 1/ n2 ) = 2s pooled /n
(L-5)
The calculated t is compared with the Student t distribution with n1 + n2 - 2
degrees of freedom.
The use of Equation L-2 to calculate t assumes that the variances of the two
groups are equal. If the variances are unequal (see Tests for Equality of
Variances below), t is computed as:
2
2
t = ( x1 - x 2 ) /
s1/ n1 + s 2/ n2
(L-6)
This statistic is compared with the Student t distribution with degrees of
freedom given by Satterthwaite's (1946) approximation:
2
( s1/ n1 + s 2/ n2 )
2
2
df = 2
(L-7)
2
2
( s1/ n1 ) / ( n1 - 1) + ( s 2/ n2 ) / ( n2 - 1)
2
This formula can result in fractional degrees of freedom, in which case one
should round df down to the nearest integer in order to use a t table. The degrees
of freedom for the t-test for unequal variances will usually be less than the
degrees of freedom for the t-test for equal variances.
Tests of Assumptions. The two-sample t-test for equal variances (and other
parametric tests such as analysis of variance) is only appropriate if:
There are independent, replicate experimental units for each treatment.
Each treatment is sampled from a normally distributed population.
Variances for both treatments are equal or similar.
The first assumption is an essential component of experimental design
(Section L.1.3.0). The second and third assumptions can be tested using the data
obtained from the experiment. Therefore, prior to conducting the t-test, tests for
normality and equality of variances should be performed. In some statistical
software packages, these tests of assumptions are done in conjunction with t-
tests or as part of data summary or screening routines that also provide means, s,
SE and various diagnostic statistics.
Outliers (extreme values) and systematic departures from a normal
distribution (e.g., a log-normal distribution) are the most common causes of
departures from normality and/or equality of variances. An appropriate
transformation will normalize many distributions. In fact, the arcsine
transformation (arcsine, in radians, of /p, where p is the survival expressed as a
proportion) is so effective, and so frequently necessary, that this Appendix
recommends applying it automatically to all survival data in the analysis of
toxicity tests. Problems with outliers can usually be solved only by using
L10
Appendix L
Statistical Methods
|
|
Privacy Statement - Press Release - Copyright Information. - Contact Us - Support Integrated Publishing |