GENERAL UNCERTAINTY ANALYSIS (cont.)

ERDC TN-DOER-I5

August 2000

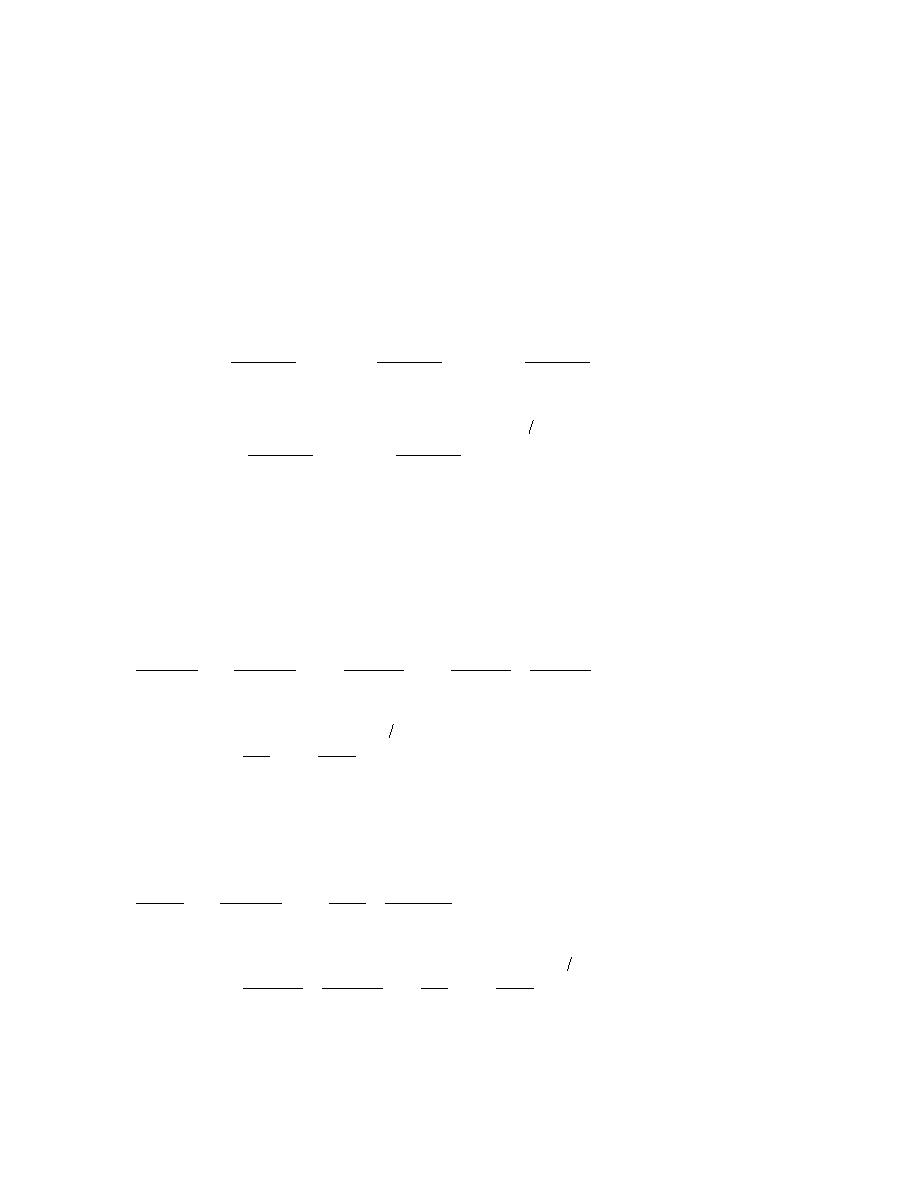
This equation is a function of five variables: the measured slurry density in the pipe (ρs), the in situ

density of the sediment (ρi), the density of the water (ρw), the flow velocity in the pipe (V), and the

pipe diameter (D). This equation can be represented in the form:

af b

VOL t = f X1, X2 , X3 , X4 , X5

(6)

which states that the in situ volumetric flow rate in the dredge pipe is a function of five independent

variables: X1, X2, X3, X4, and X5. The uncertainty in the production calculation is given by the

expression:

LMF

IJ 2 + FG

IJ 2

IJ 2 + FG

UVOLat f = G

VOL t

MNH

K H

U X1

U X2

U X3

(7)

a f IJ OP

a f IJ + FG

2 12

VOL t

K H

K PQ

U X4

U X5

which represents the square root of the sum of squares of the partial derivatives of the data reduction

equation with respect to each variable multiplied by the square of its uncertainty value (Ux).

Dividing the expression in Equation 7 by the production equation VOL(t), results in the final

uncertainty analysis expression for the flow rate of in situ material in the pipeline:

LMF Uρ I 2 + F Uρ I 2 + F Uρ - Uρ I

af G

a f NH ρs - ρw JK GH ρi - ρw JK GH ρi - ρw ρs - ρw JK

UVOL t

VOL t

(8)

2 O1 2

+ F V I + F DI P

H V K H D K PQ

This same procedural method is followed for determining the uncertainty equation for the remaining

three production equations. The final uncertainty analysis expression for the solids flow rate is:

LMF Uρ I 2 + F Uρ - Uρ I 2

af G

a f NH ρs - ρw JK GH ρm ρm - ρw JK

UM t

(9)

Uρ I F UV I 2 F 2UD I 2 O

H D K PPQ

J+

Uρ

ρi - ρw ρs - ρw K H V K