 |
||
|
|
||
| |||||||||||||||
|
|
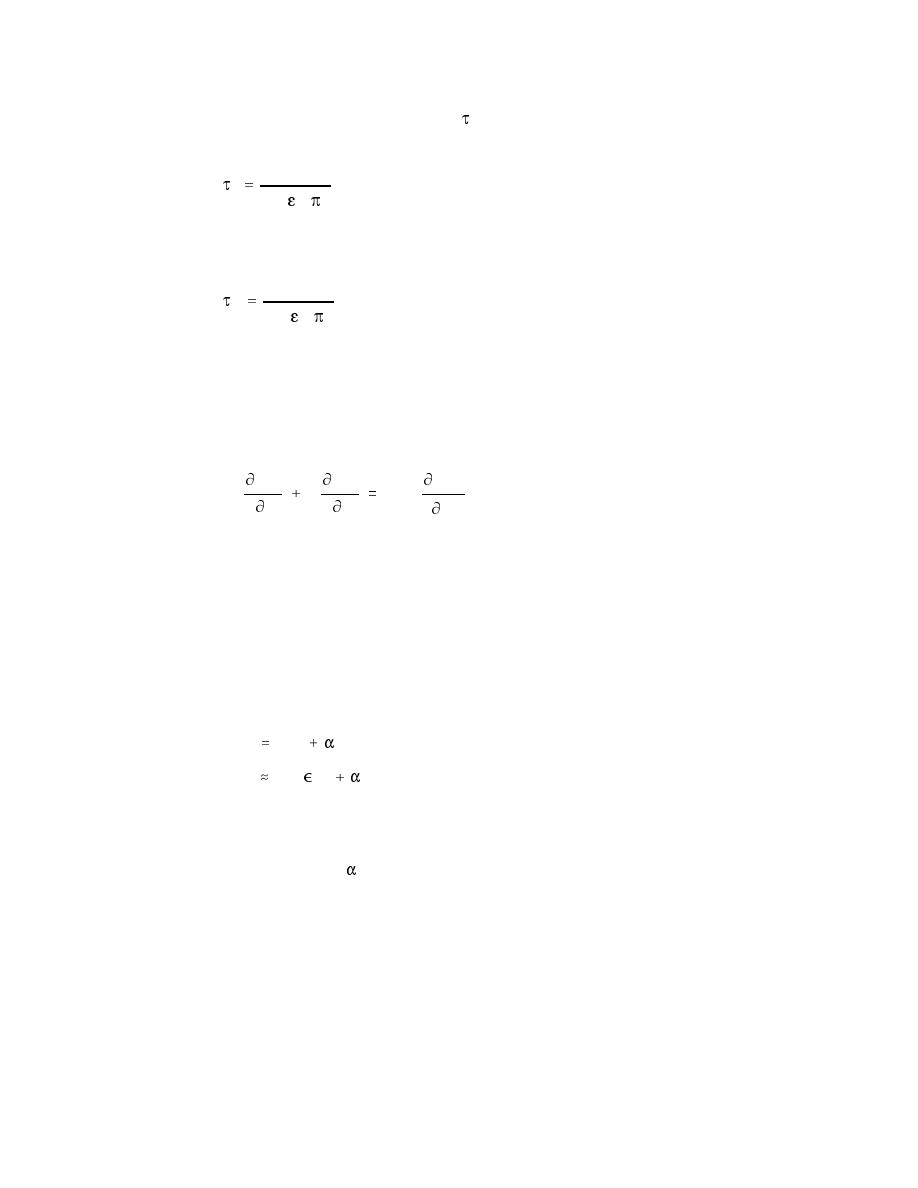 is 95 percent of its steady-state value. It is easily shown that
2
0.54 Leff Rf
(B25)
b
4/3
2
Dw
and
2
3.69 Leff Rf
(B26)
ss
4/3
2
Dw
Advective-dispersive models
When advection cannot be neglected during the operation of a cap, the basic
equation governing contaminant movement is
2
Cpw
Cpw
Cpw
Rf
(B27)
U
Ddisp
t
z
2
z
where
Cpw = contaminant concentration in pore water
U = Upw = Darcy velocity directed outward
Deff = effective diffusion/dispersion coefficient
The effective diffusion/dispersion coefficient is often modeled by a relationship
of the form (Bear 1979)
Deff
Ddisp
U
(B28)
4/3
Dw
U
The first term in this relation is associated with molecular diffusion and is again
modeled by the Millington and Quirk (1961) relation. The second term is
mechanical dispersion associated with the additional mixing due to flow vari-
ations and channeling. is the dispersivity and is typically taken to be related to
the sediment grain size (uniform sandy sediments) or travel distance (hetero-
geneous sediments). Little guidance exists for the estimation of field dispersivi-
ties for vertical flow in sediments. In uniform sandy sediments, the longitudinal
dispersivity is approximately one-half the grain diameter, while the transverse
dispersity tends to be an order of magnitude smaller (Bear 1979). Dispersion in
heterogeneous sediments would be expected to be larger than these estimates.
If the effective dispersivity can be estimated, the contaminant concentration
and flux through the cap can be estimated by solutions to Equation B27. One
B14
Appendix B Model for Chemical Containment by a Cap
|
|
Privacy Statement - Press Release - Copyright Information. - Contact Us - Support Integrated Publishing |