Models for More General Cases: Numerical Solutions

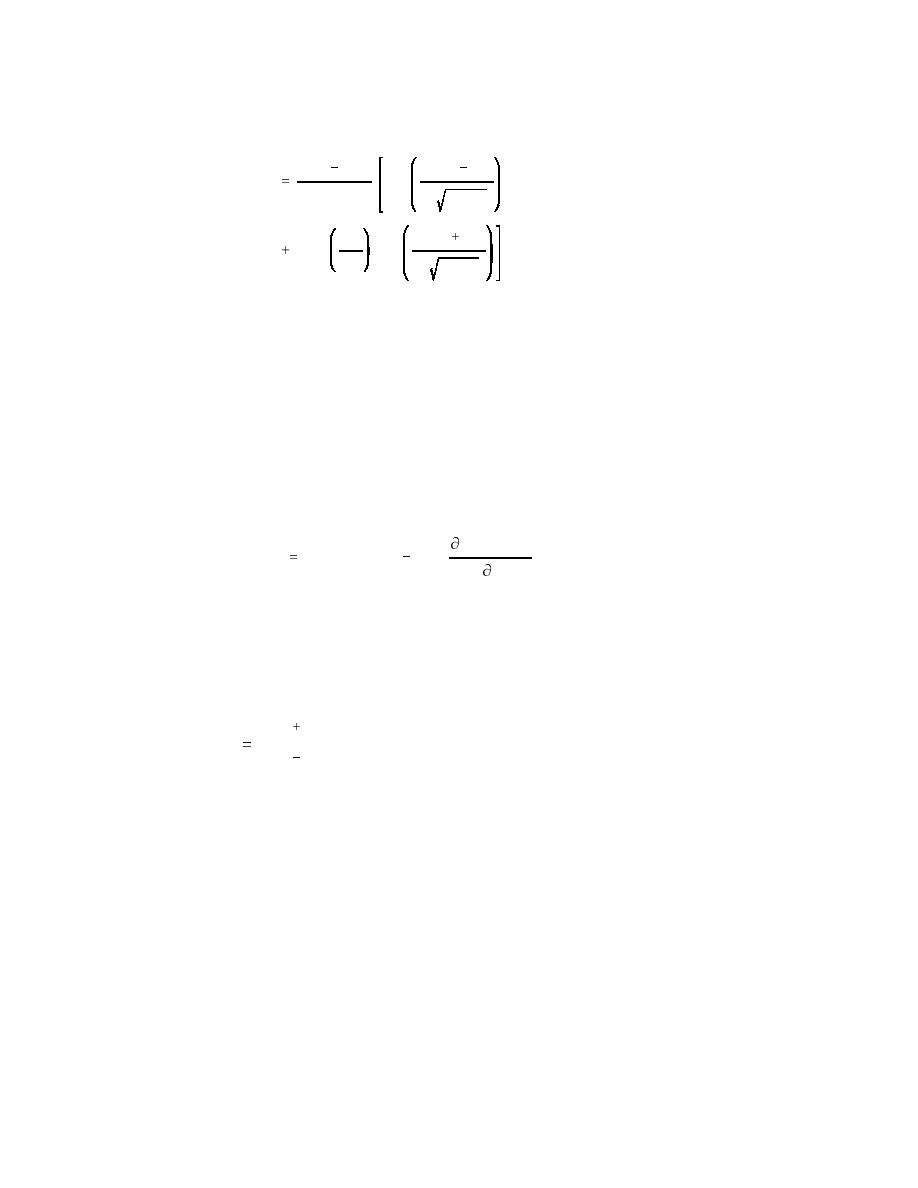The solution to Equation B27 subject to the infinite cap condition in homo-

geneous sediment is given by

Cw)

(C0

Rf z

Cpw(z,t)

erfc

2 Rf D t

(B32)

R z Ut

erfc f

exp

2 Rf D t

Here erfc represents the complementary error function that is given by 1 - erf, the

error function. The error function is a tabulated function (e.g., Thibodeaux

1996) and is commonly available in spreadsheets and computer languages. It

ranges from 0 at a value of the argument equal to zero to 1 at a value of the argu-

ment equal to infinity. The model is most useful in predicting the penetration of

the contaminant into the cap and the time until the sediment-water interface

begins to be significantly influenced by the cap, i.e., the breakthrough time. The

breakthrough time can be estimated by evaluating Equation B32 for z = Leff and

determining the time required until Cpw(Leff ,t) is equal to some fixed fraction of

the concentration in the underlying sediment; for example, until Cpw(Leff ,t) =

0.05 C0. The flux into the overlying water at any time could also be evaluated by

computing

Cpw(Leff , t)

F (Leff , t)

U Cpw(Leff , t)

Deff

(B33)

Note that Equations B32 and B33 can also be applied to conditions of mild ero-

sion or deposition on the cap. Erosion or deposition give rise to an effective

velocity directed downward with deposition and upward with erosion. Because

erosion buries or uncovers sediment and its associated contaminants, the effec-

tive velocity influencing the pore water concentration is the erosion or deposi-

tion velocity multiplied by the retardation factor.

Uerosion Rf

Erosion

Upw

(B34)

Udeposition Rf

Upw

Deposition

That is, sediment burial or deposition gives rise to a rapid burial or exposure of

contaminants as a result of the sorbed load on the sediment particles.

Models for More General Cases: Numerical

Solutions

All of the models discussed thus far assume that the concentration in the

sediment remains unchanged despite the loss of contaminant to the overlying

water. This simplification is necessary to apply the presented analytical solu-

tions but leads to overly conservative results. For example, in an advective

dominated system, Equation B29 will describe the flux to the overlying water at

long time only if depletion is not accounted for. It should be emphasized that the

B16

Appendix B Model for Chemical Containment by a Cap