Tests of Assumptions

variances. The ANOVA is conducted primarily to provide the mean square error

(MSE), which is an estimate of the pooled variance across all treatments. The

ANOVA F-statistic and its associated probability are ignored in this application.

The test statistic for the LSD is t, calculated in much the same way as for a t-

test:

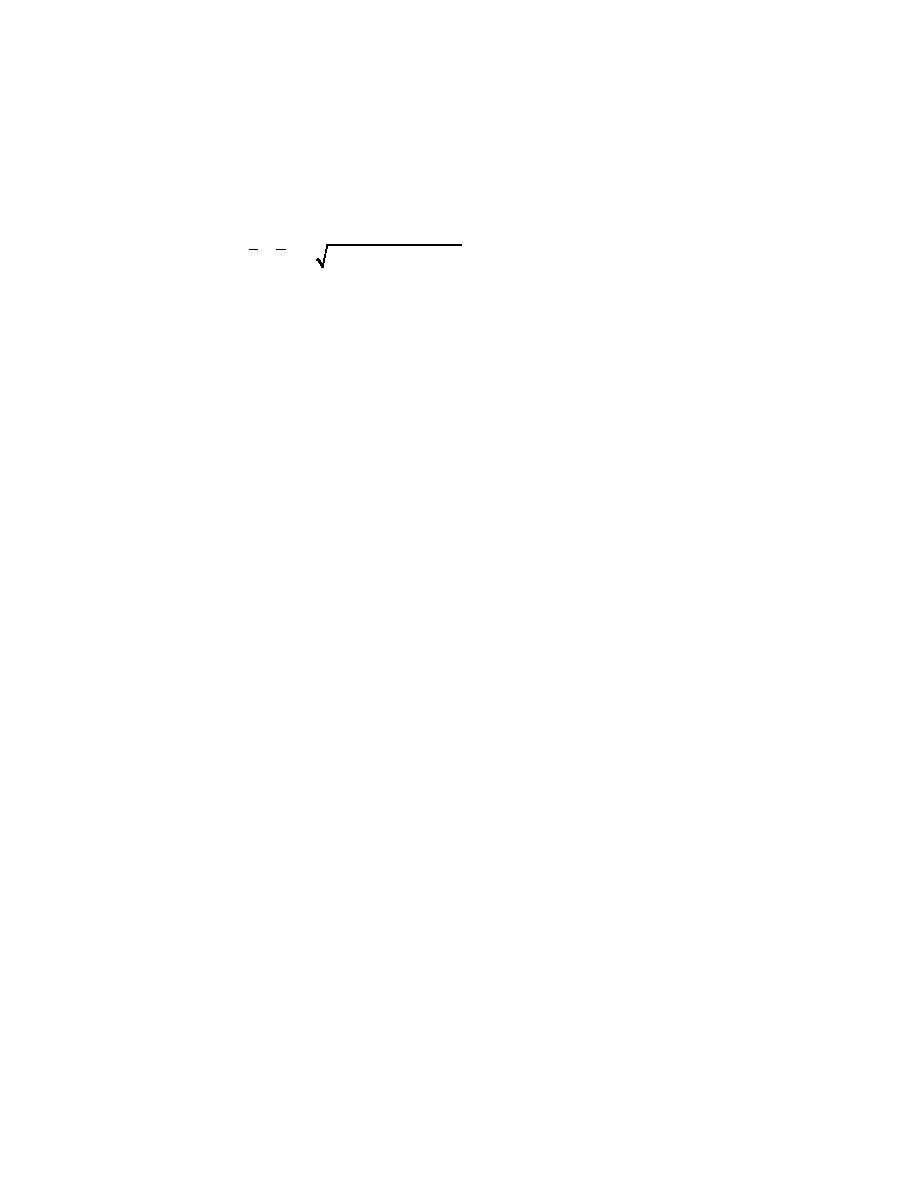
t = ( x1 - x 2 ) /

MSE (1/ n1 + 1/ n 2 )

(L-18)

This t-statistic is compared against the distribution of Student's t with N - k

degrees of freedom, where N is the total number of observations (Sn) and k is the

number of treatments including the reference. A t-statistic is computed for each

possible pair of treatments in the analysis but comparisons other than with the

reference are ignored.

The MSE can be calculated as:

MSE = Σ[ s i2( ni - 1)] / Σ( ni - 1)

(L-19)

where si2 and ni are the variance and number of replicates for the ith treatment.

The term S(ni - 1) is equivalent to N - k.

If sample sizes are equal, then (from Equation L-14):

MSE (1/ n1 + 1/ n 2 ) = 2MSE/n

(L-20)

The major advantage of using the LSD as opposed to conducting individual

two-sample t-tests comparing each dredged sediment to the reference is that the

MSE is a better estimate of the true population variance than the pooled variance

calculated from only two samples. Consequently, the LSD test is more powerful,

as reflected in the greater df for the calculated t. It also follows that a pooled

variance should only be calculated, and the LSD test conducted, if the variances

for all treatments are not significantly different from each other.

Tests of Assumptions. The Shapiro-Wilk's Test described in Sec-

tion L.2.1.1.1 can also be used to test for normality when more than two

treatments are compared. If the data are not normally distributed, even after an

appropriate transformation, then nonparametric tests should be used (see

Nonparametric Tests below).

Bartlett's Test, Levene's Test, Fmax, or Cochran's Test can be used to test for

equality of variances. When there are more than two samples, Fmax is equal to

the largest variance divided by the smallest variance. If variances are

significantly unequal, even after transformation, then each dredged sediment

should be compared with the reference using two-sample t-tests.

Nonparametric Tests. When parametric tests are not appropriate for

multiple comparisons because the normality assumption is violated, the data

should be converted to rankits, and the rankits should be tested for normality and

L28

Appendix L

Statistical Methods